Kinetic theory explains the temperature, pressure and volume of a gas in terms of the movement of molecules in the gas.
In this model gas molecules are in constant random motion. Evidence for this comes from Brownian motion where large smoke particles seem to randomly move around, this is because they are being bombarded on all sides by air molecules. the smoke particle jerks in a particular direction if the number of molecules hitting on side of it is greater than the other.
In kinetic theory the following assumptions are made about ideal gases;
- the gas contains a large number of identical molecules
- collisions between molecules are perfectly elastic as are collisions between molecules and the walls of the container
- the time for collisions to happen is negligible in comparison to the time between collisions
- molecules do not attract each other (no intermolecular forces)
- the molecules are in constant random motion
- the volume of the molecules is negligible compared to the volume of the gas (or container)
- Newton’s laws of motion can be applied to the molecules
P = pressure of the gas (Pa)
V= volume of the gas (m3)
N = the number of molecules in the gas
m = the mass of one molecule (kg)
crms = the root mean square velocity of the gas molecules (ms-1)
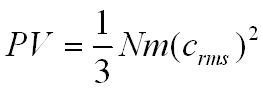
Click this link for the derivation of the above equation.
Root mean square velocity
What is the root mean square (rms) velocity of the following three velocities,
100 ms-1 150 ms-1 175 ms-1
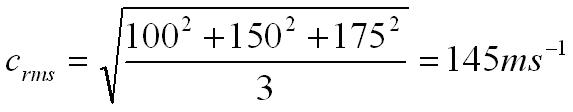
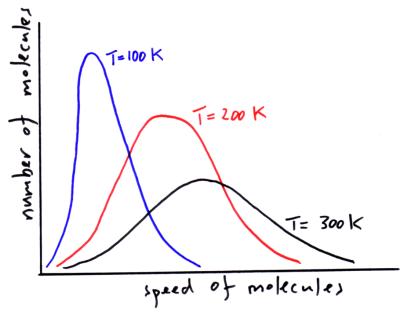
The speed of molecules in an ideal gas
In a gas there will be a range of speeds for the molecules. If the gas heats up the distribution of speeds for the molecules in the gas changes. The average speed of a molecule increases.
Kinetic Energy
The kinetic energy of a molecule in the gas is;
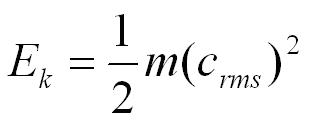
The kinetic energy of a molecule in the gas is related to the temperature of the gas by the equation below.
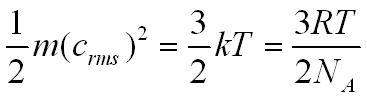
m = the mass of one molecule (kg)
crms = the root mean square velocity of the gas molecules (ms-1)pp
k = Boltzmann constant 1.38 x 10-23 JK-1
T = temperature (K)
NA = Avogadro constant 6.02 x 1023mol-1
R = molar gas constant 8.31 JK-1mol-1



